Year
A year (from Old English gēar) is the orbital period of the Earth moving around the Sun. For an observer on Earth, this corresponds to the period it takes the Sun to complete one course throughout the zodiac along the ecliptic.
In astronomy, the Julian year is a unit of time, defined as 365.25 days of 86400 SI seconds each.[1]
There is no universally accepted symbol for the year as a unit of time. The International System of Units does not propose one. A common abbreviation in international use is a (for Latin annus), in English also y or yr.
Due to the Earth's axial tilt, the course of a year sees the passing of the seasons, marked by changes in weather, hours of daylight, and consequently vegetation and fertility. In temperate and subpolar regions, generally four seasons are recognized: spring, summer, autumn and winter, astronomically marked by the Sun reaching the points of equinox and solstice, although the climatic seasons lag behind their astronomical markers. In some tropical and subtropical regions it is more common to speak of the rainy (or wet, or monsoon) season versus the dry season.
A calendar year is an approximation of the Earth's orbital period in a given calendar. A calendar year in the Gregorian calendar (as well as in the Julian calendar) has either 365 (common years) or 366 (leap years) days.
The word "year" is also used of periods loosely associated but not strictly identical with either the astronomical or the calendar year, such as the seasonal year, the fiscal year or the academic year, etc. By extension, the term year can mean the orbital period of any planet: for example, a "Martian year" is the time in which Mars completes its own orbit. The term is also applied more broadly to any long period or cycle, such as the "Great Year".[2]
Contents |
Etymology
West Saxon gear (jɛar), Anglian gēr continues Proto-Germanic *jǣram (*jē2ram). Cognates are German Jahr, Old High German jar, Old Norse ár and Gothic jer, all from a PIE *yērom "year, season". Cognates outside of Germanic are Avestan yare "year", Greek ὥρα "year, season, period of time" (whence "hour"), Old Church Slavonic jaru and Latin hornus "of this year".
Latin Annus (a 2nd declension masculine noun; annum is the accusative singular; anni is genitive singular and nominative plural; anno the dative and ablative singular) is from a PIE noun *at-no-, which also yielded Gothic aþnam "year".
Both *yē-ro- and *at-no- are based on verbal roots expressing movement, *at- and *ey- respectively, both meaning "to go" generally.
The Greek word for "year", ἔτος, is cognate with Latin vetus "old", from PIE *wetus- "year", also preserved in this meaning in Sanskrit vat-sa- "yearling (calf)" and vat-sa-ras "year".
Derived from Latin annus are a number of English words, such as annual, annuity, anniversary, etc.; per annum means "each year".
Seasonal year
A seasonal year is the time between successive recurrences of a seasonal event such as the flooding of a river, the migration of a species of bird, the flowering of a species of plant, the first frost, or the first scheduled game of a certain sport. All of these events can have wide variations of more than a month from year to year.
Calendar year
A calendar year is the time between two dates with the same name in a calendar.
A half year (one half of a year) may run from January to June, or July to December.
No astronomical year has an integer number of days or lunar months, so any calendar that follows an astronomical year must have a system of intercalation such as leap years. Financial and scientific calculations often use a 365-day calendar to simplify daily rates.
In the Julian calendar, the average length of a year is 365.25 days. In a non-leap year, there are 365 days, in a leap year there are 366 days. A leap year occurs every 4 years.
The Gregorian calendar attempts to keep the vernal equinox on or soon before March 21, hence it follows the vernal equinox year. The average length of this calendar's year is 365.2425 mean solar days (as 97 out of 400 years are leap years); this is within one ppm of the current length of the mean tropical year (365.24219 days). It is estimated that, by the year 4000, the vernal equinox will fall back by one day in the Gregorian calendar, not because of this difference, but because of the slowing down of the Earth's rotation and the associated lengthening of the sidereal day.
The Persian calendar, in use in Afghanistan and Iran, has its year begin on the day of the vernal equinox as determined by astronomical computation (for the time zone of Tehran), as opposed to using an algorithmic system of leap years.
Numbering calendar years
A calendar era is used to assign a number to individual years, using a reference point in the past as the beginning of the era. In many countries, the most common era is from the estimated date of the birth of Jesus; dates in this era are designated Anno Domini ("in the year of the Lord", abbreviated A.D.) or C.E. (common era). Other eras are also used to enumerate the years in different cultural, religious or scientific contexts.
Other annual periods
Fiscal year
A fiscal year or financial year is a 12-month period used for calculating annual financial statements in businesses and other organizations. In many jurisdictions, regulations regarding accounting require such reports once per twelve months, but do not require that the twelve months constitute a calendar year.
For example, the federal government of the U.S. has a fiscal year that starts on October 1 instead of January 1. In India the fiscal year is between April 1 and March 31. In the United Kingdom and Canada, the financial year runs from April 6 and April 1 respectively, and in Australia it runs from July 1.
Academic year
An academic year is the annual period during which a student attends an educational institution. The academic year may be divided into academic terms, such as semesters or quarters.
Some schools in the UK and USA divide the academic year into three roughly equal-length terms (called "trimesters" or "quarters" in the USA), roughly coinciding with autumn, winter, and spring. At some, a shortened summer session, sometimes considered part of the regular academic year, is attended by students on a voluntary or elective basis.
Other schools break the year into two main semesters, a first (typically August through December) and a second semester (January through May). Each of these main semesters may be split in half by mid-term exams, and each of the halves is referred to as a "quarter" (or "term" in some countries). There may also be an elective summer session and/or a short January session.
Some other schools, including some in the United States, have four marking periods. The school year in many countries starts in August or September and ends in May, June or July.
Some schools in the United States, notably Boston Latin School, may divide the year into five or more marking periods. Some state in defense of this that there is perhaps a positive correlation between report frequency and academic achievement.
There are typically 180 days of teaching each year in schools in the USA, excluding weekends and breaks, while 190 days for pupils in state schools in the United Kingdom, New Zealand and Canada.
In India the academic year normally starts from June 1 and ends on May 31. Though schools start closing from mid-March, the actual academic closure is on May 31 and in Nepal it starts from July 15.
Schools and universities in Australia typically have academic years that roughly align with the calendar year (i.e. starting in February or March and ending in October to December), as the southern hemisphere experiences summer from December to February.
Astronomical years
Julian year
The Julian year, as used in astronomy and other sciences, is a time unit defined as exactly 365.25 days. This is the normal meaning of the unit "year" (symbol "a" from the Latin annus) used in various scientific contexts. The Julian century of 36525 days and the Julian millennium of 365250 days are used in astronomical calculations. Fundamentally, expressing a time interval in Julian years is a way to precisely specify how many days (not how many "real" years), for long time intervals where stating the number of days would be unwieldy and unintuitive. By convention, the Julian year is used in the computation of the distance covered by a light-year.
In the Unified Code for Units of Measure, the symbol a (without subscript) always refers to the Julian year aj of exactly 31557600 seconds.
365.25 days of 86400 seconds = 1 a = 1 aj = 31.5576 Ms
The SI multiplier prefixes may be applied to it to form ka (kiloannum), Ma (megaannum) etc.
Sidereal, tropical, and anomalistic years
- The relations among these are considered more fully in Axial precession (astronomy).
Each of these three years can be loosely called an 'astronomical year'.
The sidereal year is the time taken for the Earth to complete one revolution of its orbit, as measured against a fixed frame of reference (such as the fixed stars, Latin sidera, singular sidus). Its average duration is 365.256363004 mean solar days (365 d 6 h 9 min 9.76 s) (at the epoch J2000.0 = January 1, 2000, 12:00:00 TT).[3]
The tropical year is the period of time for the ecliptic longitude of the Sun to increase by 360 degrees. Since the Sun's ecliptic longitude is measured with respect to the equinox, the tropical year comprises a complete cycle of the seasons; because of the economic importance of the seasons, the tropical year is the basis of most calendars. The tropical year is often defined as the time between southern solstices, or between northward equinoxes. Because of the Earth's axial precession, this year is about 20 minutes shorter than the sidereal year. The mean tropical year is approximately 365 days, 5 hours, 48 minutes, 45 seconds[4] (= 365.24219 days).
The anomalistic year is the time taken for the Earth to complete one revolution with respect to its apsides. The orbit of the Earth is elliptical; the extreme points, called apsides, are the perihelion, where the Earth is closest to the Sun (January 3 in 2011), and the aphelion, where the Earth is farthest from the Sun (July 4 in 2011). The anomalistic year is usually defined as the time between perihelion passages. Its average duration is 365.259636 days (365 d 6 h 13 min 52.6 s) (at the epoch J2011.0).[5]
If Earth moved in an ideal Kepler orbit, i.e. a perfect ellipse with the Sun fixed at one focus, each kind of year would always have the same duration, and the sidereal and anomalistic years would be equal. Because of perturbations by the gravity of other planets, Earth's motion varies slightly, causing the sidereal and tropical years to vary in length by about 25 minutes (see table below). Both are affected in the same way, so that the sidereal year is consistently 20 minutes longer than the tropical year, provided that they are measured in the same way.
| Winter solstice (Atomic time) | Deviation of the following year's duration from the mean value 365.24219 SI days |
|---|---|
| 2007-12-22 06:04:04.2 | +10.51 minutes |
| 2008-12-21 12:03:19.7 | -11.86 minutes |
| 2009-12-21 17:40:13.2 | +15.91 minutes |
| 2010-12-21 23:44:53.2 | -11.94 minutes |
| 2011-12-22 05:21:41.8 | +3.58 minutes |
| 2012-12-21 11:14:01.9 | +2.85 minutes |
| 2013-12-21 17:05:38.3 | +0.86 minutes |
| 2014-12-21 22:55:15.2 | +0.48 minutes |
An example of a year that will have a duration exceeding the average value of 365.24219 SI days with as much as 24.23 minutes is the one that will begin at winter solstice December 21, 2042 17:47:45.5 (Atomic time).
Draconic year
The draconic year, draconitic year, eclipse year, or ecliptic year is the time taken for the Sun (as seen from the Earth) to complete one revolution with respect to the same lunar node (a point where the Moon's orbit intersects the ecliptic). This period is associated with eclipses: these occur only when both the Sun and the Moon are near these nodes; so eclipses occur within about a month of every half eclipse year. Hence there are two eclipse seasons every eclipse year. The average duration of the eclipse year is
- 346.620075883 days (346 d 14 h 52 min 54 s) (at the epoch J2000.0).
This term is sometimes erroneously used for the draconic or nodal period of lunar precession, that is the period of a complete revolution of the Moon's ascending node around the ecliptic: 18.612815932 Julian years (6798.331019 days; at the epoch J2000.0).
Full moon cycle
The full moon cycle is the time for the Sun (as seen from the Earth) to complete one revolution with respect to the perigee of the Moon's orbit. This period is associated with the apparent size of the full moon, and also with the varying duration of the synodic month. The duration of one full moon cycle is:
- 411.78443029 days (411 d 18 h 49 min 34 s) (at the epoch J2000.0).
Lunar year
The lunar year comprises twelve full cycles of the phases of the Moon, as seen from Earth. It has a duration of approximately 354.37 days.
Vague year
The vague year, from annus vagus or wandering year, is an integral approximation to the year equaling 365 days, which wanders in relation to more exact years. Typically the vague year is divided into 12 schematic months of 30 days each plus 5 epagomenal days. The vague year was used in the calendars of Ancient Egypt, Iran, Armenia and in Mesoamerica among the Aztecs and Maya.[6]
Heliacal year
A heliacal year is the interval between the heliacal risings of a star. It differs from the sidereal year for stars away from the ecliptic due mainly to the precession of the equinoxes.
Sothic year
The Sothic year is the interval between heliacal risings of the star Sirius. It is presently less than the sidereal year and its duration is very close to the mean Julian year of 365.25 days.
Gaussian year
The Gaussian year is the sidereal year for a planet of negligible mass (relative to the Sun) and unperturbed by other planets that is governed by the Gaussian gravitational constant. Such a planet would be slightly closer to the Sun than Earth's mean distance. Its length is:
- 365.2568983 days (365 d 6 h 9 min 56 s).
Besselian year
The Besselian year is a tropical year that starts when the (fictitious) mean Sun reaches an ecliptic longitude of 280°. This is currently on or close to January 1. It is named after the 19th century German astronomer and mathematician Friedrich Bessel. The following equation can be used to compute the current Besselian epoch (in years):[7]
- B = 1900.0 + (Julian dateTT − 2415020.31352) / 365.242198781
The TT subscript indicates that for this formula, the Julian date should use the Terrestrial Time scale, or its predecessor, ephemeris time.
Variation in the length of the year and the day
The exact length of an astronomical year changes over time. The main sources of this change are:
- The precession of the equinoxes changes the position of astronomical events with respect to the apsides of Earth's orbit. An event moving toward perihelion recurs with a decreasing period from year to year; an event moving toward aphelion recurs with an increasing period from year to year (though this effect does not change the average value of the length of the year).
- Each planet's movement is perturbed by the gravity of every other planet.
- Tidal drag between the Earth and the Moon and Sun increases the length of the day and of the month (by transferring angular momentum from the rotation of the Earth to the revolution of the Moon); since the apparent mean solar day is the unit with which we measure the length of the year in civil life, the length of the year appears to change. Tidal drag in turn depends on factors such as post-glacial rebound and sea level rise.
- Changes in the effective mass of the Sun, caused by solar wind and radiation of energy generated by nuclear fusion and radiated by its surface, will affect the Earth's orbital period over a long time (approximately an extra 1.25 microsecond per year).[8]
- The Poynting–Robertson effect shortens the year by about 30 nanoseconds per year.
- Gravitational radiation shortens the year by about 165 attoseconds per year.[9]
Summary
- 346.62 days: a draconitic year.
- 353, 354 or 355 days: the lengths of common years in some lunisolar calendars.
- 354.37 days (12 lunar months): the average length of a year in lunar calendars, notably the Muslim calendar.
- 365 days: a vague year and a common year in many solar calendars.
- 365.24219 days: a mean tropical year (rounded to five decimal places) for the epoch 2000.
- 365.2424 days: a vernal equinox year (rounded to four decimal places) for the epoch 2000.
- 365.2425 days: the average length of a year in the Gregorian calendar.
- 365.25 days: the average length of a year in the Julian calendar.
- 365.2564 days: a sidereal year.
- 366 days: a leap year in many solar calendars.
- 383, 384 or 385 days: the lengths of leap years in some lunisolar calendars.
- 383.9 days (13 lunar months): a leap year in some lunisolar calendars.
An average Gregorian year is 365.2425 days = 52.1775 weeks = 8765.82 hours = 525949.2 minutes = 31556952 seconds (mean solar, not SI).
A common year is 365 days = 8760 hours = 525600 minutes = 31536000 seconds.
A leap year is 366 days = 8784 hours = 527040 minutes = 31622400 seconds.
The 400-year cycle of the Gregorian calendar has 146097 days and hence exactly 20871 weeks.
See also Leap seconds and other aspects of the Gregorian calendar.
Symbol
There is no universally accepted symbol for the year as a unit of time. The International System of Units does not propose one. NIST SP811[10] and ISO 80000-3:2006[11] suggest the symbol a is taken from the Latin word annus.[12] In English, the abbreviations y or yr are sometimes used, specifically in geology and paleontology, where kyr, myr, byr (thousands, millions, and billions of years, respectively) and similar abbreviations are used to denote intervals of time remote from the present.[12][13][14]
Symbol a
NIST SP811[15] and ISO 80000-3:2006[16] suggest the symbol a (in the International System of Units, although a is also the symbol for the are, the unit of area used to measure land area, but context is usually enough to disambiguate). In English, the abbreviations y and yr are also used.[12] [13] [14]
The Unified Code for Units of Measure[17] disambiguates the varying symbologies of ISO 1000, ISO 2955 and ANSI X3.50 [1] by using
- ar for are and:
- at = a_t = 365.24219 days for the mean tropical year
- aj = a_j = 365.25 days for the mean Julian year
- ag = a_g = 365.2425 days for the mean Gregorian year
- a = 1 aj year (without further qualifier)
A definition jointly adopted by the International Union of Pure and Applied Chemistry and the International Union of Geological Sciences is to use annus, with symbol a, for year, defined as the length of the tropical year in the year 2000:[18][19]
- a = 365.24219265 days = 31556925.445 seconds
The notation has proved controversial; it conflicts with an earlier convention among geoscientists to use a specifically for "years ago", and y or yr for a one-year time period.[19]
SI prefix multipliers
- ka (for kiloannum), is a unit of time equal to one thousand (103) years.
- Ma (for megaannum), is a unit of time equal to one million (106) years. It is commonly used in scientific disciplines such as geology, paleontology, and celestial mechanics to signify very long time periods into the past or future. For example, the dinosaur species Tyrannosaurus rex was abundant approximately 65 Ma (65 million years) ago (ago may not always be mentioned; if the quantity is specified while not explicitly discussing a duration, one can assume that "ago" is implied; the alternative but deprecated "mya" unit includes "ago" explicitly.). In astronomical applications, the year used is the Julian year of precisely 365.25 days. In geology and paleontology, the year is not so precise and varies depending on the author.
- Ga (for gigaannum), is a unit of time equal to 109 years (one billion on the short scale, one milliard on the long scale). It is commonly used in scientific disciplines such as cosmology and geology to signify extremely long time periods in the past. For example, the formation of the Earth occurred approximately 4.57 Ga (4.57 billion years) ago.
- Ta (for teraannum), is a unit of time equal to 1012 years (one trillion on the short scale, one billion on the long scale). It is an extremely long unit of time, about 70 times as long as the age of the universe. It is the same order of magnitude as the expected life span of a small red dwarf star.
- Pa (for petaannum), is a unit of time equal to 1015 years (one quadrillion on the short scale, one billiard on the long scale). The half-life of the nuclide cadmium-113 is about 8 Pa. This symbol coincides with that for the pascal without a multiplier prefix, though both are infrequently used and context will normally be sufficient to distinguish time from pressure values.
- Ea (for exaannum), is a unit of time equal to 1018 years (one quintillion on the short scale, one trillion on the long scale). The half-life of tungsten-180 is 1.8 Ea.[20]
Symbols y and yr
In astronomy, geology, and paleontology, the abbreviation yr for "years" and ya for "years ago" are sometimes used, combined with prefixes for "thousand", "million", or "billion".[13][21] They are not SI units, using y to abbreviate English year, but following ambiguous international recommendations, use either the standard English first letters as prefixes (t, m, and b) or metric prefixes (k, M, and G) or variations on metric prefixes (k, m, g). These abbreviations include:
| SI-prefixed equivalent | order of magnitude | |
| kyr | "ka" | * Thousands forms |
| myr | "Ma" | * Millions forms |
| byr | "Ga" | * Billions forms |
| tya or kya | "ka ago" |
|
| mya | "Ma ago" |
|
| bya or gya | "Ga ago" |
|
Use of "mya" and "bya" is deprecated in modern geophysics, the recommended usage being "Ma" and "Ga" for dates Before Present, but "m.y." for the duration of epochs.[13][14] This ad hoc distinction between "absolute" time and time intervals is somewhat controversial amongst members of the Geological Society of America.[23]
Note that on graphs using "ya" units on the horizontal axis time flows from right to left, which may seem counter-intuitive. If the "ya" units are on the vertical axis, time flows from top to bottom which is probably easier to understand than conventional notation.
"Great years"
Equinoctial cycle
The Great year, or Equinoctial cycle corresponds to a complete revolution of the equinoxes around the ecliptic. Its length is about 25,700 years, and cannot be determined precisely as the precession speed is variable.
Galactic year
The Galactic year is the time it takes Earth's solar system to revolve once around the galactic center. It comprises roughly 230 million Earth years.[24]
See also
- 2012
- Astronomical year numbering
- ISO 8601: standard for representation of dates and times
- Jēran
- List of calendars
- List of years
- Man-hour
- Orders of magnitude (time)
- Unit of time
References
Notes
- ^ International Astronomical Union "SI units" accessed February 18, 2010. (See Table 5 and section 5.15.) Reprinted from George A. Wilkins & IAU Commission 5, "The IAU Style Manual (1989)" (PDF file) in IAU Transactions Vol. XXB
- ^ OED, s.v. "year", entry 2.b.: "transf. Applied to a very long period or cycle (in chronology or mythology, or vaguely in poetic use)."
- ^ International Earth Rotation and Reference System Service. (2010).IERS EOP PC Useful constants.
- ^ Astronomical Almanac for the Year 2011. Washington and Taunton: U.S. Government Printing Office and the U.K. Hydrographic Office. 2009. p. M18 (Glossary). http://asa.usno.navy.mil/SecM/Glossary.html#y.
- ^ Astronomical Almanac for the Year 2011. Washington and Taunton: U.S. Government Printing Office and the U.K. Hydrographic Office. 2009. pp. A1, C2.
- ^ Calendar Description and Coordination Maya World Studies Center
- ^ Astronomical Almanac for the Year 2010. Washington and Taunton: U.S. Government Printing Office and the U.K. Hydrographic Office. 2008. p. B3.
- ^ Solar mass is ~2×1030 kg, decreasing at ~5×109 kg/s, or ~8×10−14 solar mass per year. The period of an orbiting body is proportional to
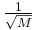 , where M is the mass of the primary.
, where M is the mass of the primary. - ^ ~300 W of radiation produces ~9.5×109 J orbital energy decrease per year; this varies as 1/R, and period varies as R1.5
- ^ Ambler Thompson, Barry N. Taylor (2008) (PDF). Special Publication 811: Guide for the Use of the International System of Units (SI). National Institute of Standards and Technology (NIST). http://physics.nist.gov/Document/sp811.pdf.
- ^ "ISO 80000-3:2006, Quantities and units – Part 3: Space and time". Geneva, Switzerland: International Organization for Standardization. 2006. http://www.iso.org/iso/iso_catalogue/catalogue_tc/catalogue_detail.htm?csnumber=31888.
- ^ a b c Russ Rowlett. "Units: A". How Many? A Dictionary of Units of Measurement. University of North Carolina. http://www.unc.edu/~rowlett/units/dictA.html. Retrieved January 9, 2009.
- ^ a b c d "AGU Editorial Style Guide for Authors". American Geophysical Union. September 21, 2007. Archived from the original on 2008-07-14. http://web.archive.org/web/20080714134306/http://www.agu.org/pubs/style_guide_intro.html. Retrieved 2009-01-09.
- ^ a b c North American Commission on Stratigraphic Nomenclature (November 2005). "North American Stratigraphic Code". The American Association of Petroleum Geologists Bulletin 89 (11): 1547–1591. http://ngmdb.usgs.gov/Info/NACSN/Code2/code2.html#Article13.
- ^ Ambler Thompson, Barry N. Taylor (2008). "Special Publication 811 – Guide for the Use of the International System of Units (SI)". National Institute of Standards and Technology (NIST). para 8.1. http://physics.nist.gov/Document/sp811.pdf.
- ^ "ISO 80000-3:2006, Quantities and units". Geneva: International Organization for Standardization. 2006. Part 3: Space and time. http://www.iso.org/iso/iso_catalogue/catalogue_tc/catalogue_detail.htm?csnumber=31888.
- ^ Gunther Schadow, Clement J. McDonald. "Unified Code for Units of Measure". http://aurora.rg.iupui.edu/ucum.
- ^ Norman E. Holden, Mauro L. Bonardi1, Paul De Bièvre, Paul R. Renne, and Igor M. Villa (2011). "IUPAC-IUGS common definition and convention on the use of the year as a derived unit of time (IUPAC Recommendations 2011)". Pure and Applied Chemistry 83 (5): 1159–1162. doi:10.1351/PAC-REC-09-01-22.
- ^ a b Celeste Biever (April 27, 2011). "Push to define year sparks time war". New Scientist. http://www.newscientist.com/article/dn20423-push-to-define-year-sparks-time-war.html. Retrieved April 28, 2011.
- ^ F. A. Danevich et al. (2003). "α activity of natural tungsten isotopes". Phys. Rev. C 67: 014310. arXiv:nucl-ex/0211013. doi:10.1103/PhysRevC.67.014310.
- ^ North American Commission on Stratigraphic Nomenclature. "Article 13 (c)". North American Stratigraphic Code. http://ngmdb.usgs.gov/Info/NACSN/Code2/code2.html#Article13. "(c) Convention and abbreviations. – The age of a stratigraphic unit or the time of a geologic event, as commonly determined by numerical dating or by reference to a calibrated time-scale, may be expressed in years before the present. The unit of time is the modern year as presently recognized worldwide. Recommended (but not mandatory) abbreviations for such ages are SI (International System of Units) multipliers coupled with "a" for annum: ka, Ma, and Ga for kilo-annum (103 years), Mega-annum (106 years), and Giga-annum (109 years), respectively. Use of these terms after the age value follows the convention established in the field of C-14 dating. The "present" refers to 1950 AD, and such qualifiers as "ago" or "before the present" are omitted after the value because measurement of the duration from the present to the past is implicit in the designation. In contrast, the duration of a remote interval of geologic time, as a number of years, should not be expressed by the same symbols. Abbreviations for numbers of years, without reference to the present, are informal (e.g., y or yr for years; my, m.y., or m.yr. for millions of years; and so forth, as preference dictates). For example, boundaries of the Late Cretaceous Epoch currently are calibrated at 63 Ma and 96 Ma, but the interval of time represented by this epoch is 33 m.y."
- ^ Bradford M. Clement (April 8, 2004). "Dependence of the duration of geomagnetic polarity reversals on site latitude". Nature 428 (6983): 637–40. doi:10.1038/nature02459. PMID 15071591.
- ^ "Time Units". Geological Society of America. http://www.geosociety.org/TimeUnits/. Retrieved February 17, 2010.
- ^ "Science Bowl Questions, Astronomy, Set 2". Science Bowl Practice Questions. Oak Ridge Associated Universities. 2009. http://www.orau.gov/SCIENCEBOWL/teams/files/astrset2.pdf. Retrieved December 9, 2009.
Further reading
- Fraser, Julius Thomas (1987). Time, the Familiar Stranger (illustrated ed.). Amherst: University of Massachusetts Press. ISBN 0870235761. OCLC 15790499.
- Whitrow, Gerald James (2003). What is Time?. Oxford: Oxford University Press. ISBN 0198607814. OCLC 265440481.
|
|||||||||||||||||||||||||||||||||||||
|
||||||||||||||||||||||||||||||||